Explore the World's Best Ideas
Join today and uncover 100+ curated journeys from 50+ topics. Unlock access to our mobile app with extensive features.
Calculating The Golden Ratio
There is a lot of math behind how the Golden Ratio is calculated. But designers, artists, and photographers aren’t usually high-level mathematicians. To benefit the algebraically disinclined, let’s keep it simple.
The Ratio is approximately 1.618 to 1. It’s approximate because it is an irrational number that continues with an infinite number of decimal places. In math, the number is referred to by the Greek letter φ or Phi.
38
318 reads
The History
The Ratio was first described by the ancient Greek mathematicians Phidias, Plato, and Euclid, as early as c. 450 B.C. It has been studied and refined for two and a half millennia.
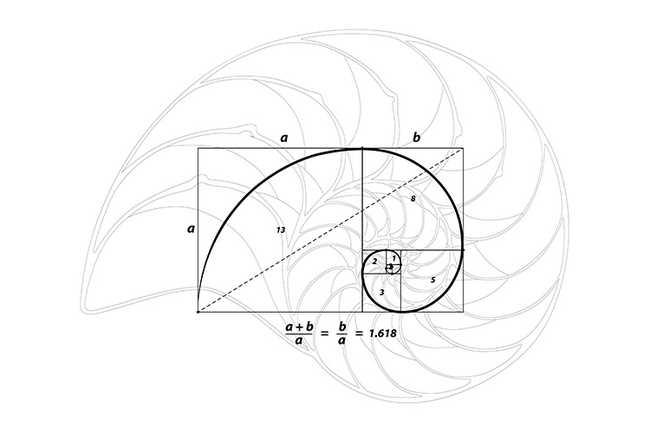
The Ratio is also closely related to the Fibonacci sequence. This mathematical pattern shows numbers that are added together to make the next number. The first numbers of the sequence are 0, 1, 1, 2, 3, 5, 8, 13, 21, etc.
38
211 reads
The Mystery of Imperfection
One of the most common arguments against the importance of Phi is that, while Phi can be applied to many things in nature, careful analysis shows that it is seldom mathematically perfect. When the spirals of a nautilus shell are measured, their ratio to one another is not exactly 1.618.
Famous pieces of artwork and architecture fail to stand up to careful study as well. This is true from ancient pyramids and famous buildings from ancient Greece to the Mona Lisa and other artworks by the masters.
37
195 reads
The Rule Of Thirds
It has often been claimed that the Rule of Thirds in photography is a simplification of Phi. It creates roughly the same result while being easier to apply in the field or on the go. Does the Rule of Thirds help photographers create more captivating images? It does.
Does it have to be applied perfectly every time? No, you can get great results and beautiful photos by using it as a starting point and modifying it as you see fit.
37
174 reads
Mathematically Inaccurate But Beautiful
Whether we realize it or not, humans can’t help but find objects that conform to Phi as intrinsically beautiful.
As artists and creators, we can use this predisposition to our benefit. By incorporating the Ratio in our design and photography, we can improve the quality and beauty of our work.
38
171 reads
Applying The Golden Ratio
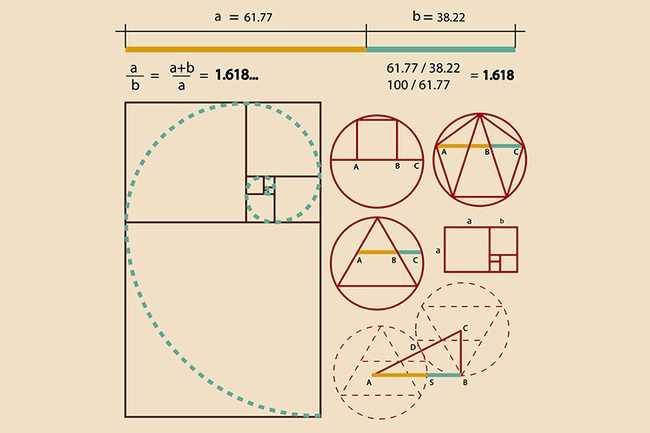
In algebra, it is usually shown with the variables a and b.
But when working with visual arts, it’s easiest to apply it geometrically. The most common example is the use of rectangles. A Golden Rectangle is one whose short edge is 1, while the long side is 1.618. The rectangle can then be divided into itself by the magic number 1.618 an infinite number of times.
A common extrapolation of this is the Golden Spiral. By looking at the image of the slowly diminishing rectangles, the dimensions of a spiral shape can be drawn.
37
138 reads
The Nature Has It Already
The Golden Ratio is a naturally occurring phenomenon that can be observed in the spirals of a seashell, rainbands of a hurricane, petals of a flower, or the leaves of a plant. It’s only natural for artists to mimic the beauty of nature.
37
145 reads
Golden Ratio in Design
In design, layouts are the perfect place to start applying the Ratio. Two-column layouts are extremely common. But weighing the columns differently adds a dynamic flow to any publications. Webpages, in particular, use the sidebar concept to apply a dynamic, weighted feel that works naturally.
But what should the dimensions be? The units of measurement do not matter. The larger edge of the larger rectangle should be 1.618 times the length of the edge of the smaller rectangle.
39
143 reads
Logo Design With Golden Ratio
Many iconic logos can be distilled down to the ratio. By using 1:1.618 to all manner of shapes, cutouts, fills, and patterns, the symmetry of design can really come together. Search online, and you can find some excellent analyses of how some of the most iconic corporate brands benefit from the use of the Ratio in their designs.
It’s essential to realize that the Ratio can be repeated in a work several times over.
37
113 reads
Golden Ratio in Photography
Many photographers use the Rule of Thirds as a simplified form of the Ratio. In the Rule of Thirds, you simply divide the frame into one-third sections vertically and horizontally.
Important elements are placed at the intersections of the lines. The lines can also be used in the photo itself. Horizons in landscapes are commonly placed on a horizontal one-thirds line.
37
115 reads
Discovering New Places In Nature Using The Golden Ratio
Another use of Phi in photography is to capture objects that already possess it. Plant leaves, distant spiral galaxies, flower petals, and seashells are alluring objects that make great subjects.
The challenge is to recognize how the golden spiral plays a part in making that beauty and to capture it in an image. What can you do to emphasize the spiral and communicate that beauty to your viewers? Seek out objects that already use the Ratio within and leverage this to your advantage.
37
103 reads
IDEAS CURATED BY
Michael Fitzgerald's ideas are part of this journey:
Learn more about product with this collection
Essential product management skills
How to work effectively with cross-functional teams
How to identify and prioritize customer needs
Related collections
Similar ideas
4 ideas
What is the Fibonacci sequence?
sciencefocus.com
2 ideas
The 8 Elements of Composition in Art
thoughtco.com
6 ideas
Read & Learn
20x Faster
without
deepstash
with
deepstash
with
deepstash
Personalized microlearning
—
100+ Learning Journeys
—
Access to 200,000+ ideas
—
Access to the mobile app
—
Unlimited idea saving
—
—
Unlimited history
—
—
Unlimited listening to ideas
—
—
Downloading & offline access
—
—
Supercharge your mind with one idea per day
Enter your email and spend 1 minute every day to learn something new.
I agree to receive email updates