Hempel’s Ravens Paradox | Platonic Realms
Curated from: platonicrealms.com
Ideas, facts & insights covering these topics:
6 ideas
·988 reads
11
Explore the World's Best Ideas
Join today and uncover 100+ curated journeys from 50+ topics. Unlock access to our mobile app with extensive features.
Hempel’s Ravens Paradox
The philosopher Carl G. Hempel, in his 1965 essay “Studies in the Logic of Confirmation,” brought to light a central paradox in the scientific method as it is commonly understood.
This is called Hempel’s Ravens Paradox, and it deals with inductive reasoning, which is the first step in any scientific method: Observing and forming a hypothesis.
15
218 reads
Inductive Reasoning
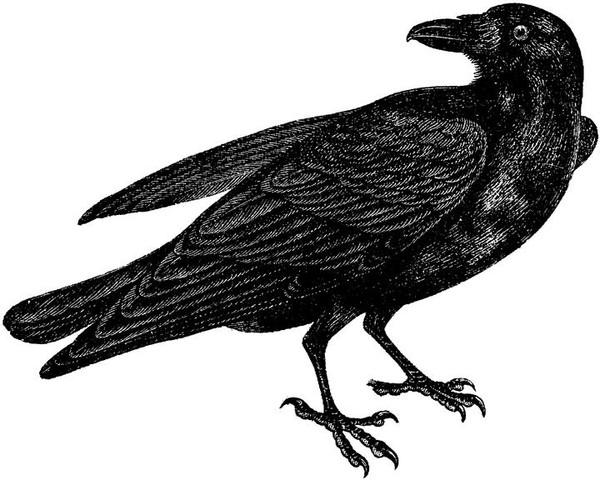
Suppose you see a raven, and you note that it is black. “Hmm,” you say, “that raven was black.” Sometime later you notice a couple more ravens, and they also are black. “What a coincidence,” you remark, “those ravens are black too.” Time goes by and you see many more ravens. And it happens that all the ravens you see are black. “This is beyond coincidence,” you might reasonably think, and with the instincts of a good and observant scientist you form a hypothesis: All ravens are black.
14
175 reads
The Limitation
There are always limits to an experimental apparatus, even if the apparatus is just a matter of observing as many ravens as possible to check their colour. Nonetheless, we feel justified in saying that each new observation of a black raven tends to confirm the hypothesis, and in time, if no green or blue or otherwise non-black ravens are observed, our hypothesis will eventually come to have the status of natural law.
14
153 reads
The Flaw In Our Logic
Logically put, our hypothesis “all ravens are black” has the form of a conditional, that is, a statement of the form “if A then B.” In short, we are saying that if a given object is a raven, then that object is black. According to the laws of logic, a conditional is equivalent to its contrapositive. That is, a statement of the form “if A then B” is equivalent to the statement “if not B then not A.” This rule of logic is incontrovertible.
15
153 reads
The Illogical Conclusion
Our hypothesis “all ravens are black” therefore has the equivalent form “all non-black things are non-ravens,” or more precisely, “if an object isn't black then it is not a raven.” Consequently, if every sighting of a black raven confirms our hypothesis, then every sighting of a non-black non-raven equally confirms our hypothesis.
14
155 reads
The Bottom Line
Very well, you might say, but maybe every sighting of a non-black non-raven really does confirm, even if only to an infinitesimal degree, the hypothesis that all ravens are black. After all, if we could, somehow, check every non-black object in the universe, and if none of them were ravens, our statement that all ravens are black would be proved.
15
134 reads
IDEAS CURATED BY
Charlie 's ideas are part of this journey:
Learn more about problemsolving with this collection
How to set clear objectives
How to follow up after a meeting
How to manage time effectively
Related collections
Similar ideas
4 ideas
3 ideas
The hardest question ever asked: What is truth?
bigthink.com
6 ideas
Cherry Picking: When People Ignore Evidence that They Dislike
effectiviology.com
Read & Learn
20x Faster
without
deepstash
with
deepstash
with
deepstash
Personalized microlearning
—
100+ Learning Journeys
—
Access to 200,000+ ideas
—
Access to the mobile app
—
Unlimited idea saving
—
—
Unlimited history
—
—
Unlimited listening to ideas
—
—
Downloading & offline access
—
—
Supercharge your mind with one idea per day
Enter your email and spend 1 minute every day to learn something new.
I agree to receive email updates